Non-changing current with C-EMF

[XRZ]

Im just trying to answer a question out of curiosity.
I think the idea of the secondary conductor would not changing anything(but add more current in opposition). I don't see how by changing the wires it would actually cancel the other's induced EMF when there is power supply in the circuit. If the power supply is connected to the circuit they would cancel.

 

[Arouse1973]

Sorry I dont understand this can you explain in more detail
Adam

 

[XRZ]

What didn't you understand? I said a lot

 

[Arouse1973]

The whole lot. Sorry.
Adam

 

[XRZ]

Well, I was wondering about Merlin3189's post:

2- The obvious way to hold the current constant is, as Arouse said, to balance the circuit. For example have a second identical slab of copper in the same field but wired into the circuit in the reverse direction. The emfs will exactly balance out and leave the current constant.

I'm questioning the possibility of such an example , and I think the addition of another conductor would actually increase the induced current in opposition to the current source. I've attached some diagrams to show that.

 

[(*steve*)]

Yes, that has come up before. People think back EMF is a bad thing. It is not, electric motors would not be practical without it.

Worse than that, if you counter the magnetic field, the motor would both (a) always draw the stall current, and (b) not work.

 

[(*steve*)]

and I think the addition of another conductor would actually increase the induced current in opposition

And here is the reason not to carry on conversations as PM's. Someone will now repeat what I told you.

 

[XRZ]

From your PM:

The main issue that I see is that you absolutely want the current to vary in an electric motor.

If you always had the stall current flowing, even when the motor was running with no load, you would be very sad.

No. This whole thread had nothing to do with an electric motor, I just used it to an example to justify why I came up with my question, and yet we dove into that for some reason.

And here is the reason not to carry on conversations as PM's. Someone will now repeat what I told you.

That voltage can't be cancelled?

 

[(*steve*)]

No. This whole thread had nothing to do with an electric motor, I just used it to an example to justify why I came up with my question, and yet we dove into that for some reason.

In that case I *still* don't know what it's about.

That voltage can't be cancelled?

Go back and read my PM's. If you have any questions, post your PMs to me and my responses here. There is no reason for people to do the same work more than once.

 

[Merlin3189]

What I had in mind would look like the attached image.
I've represented your Copper slab as lumped properties, resistance to represent the Ohmic resistance and pure inductance L1 to represent the effect of a magnetic field generating an emf. In the second circuit L2 and L3 represent this effect in two slabs (I could not find an easy way to label one as L1 again.) If the same field passes through both inductances, then each should generate exactly the same emf. Since they are connected in opposite senses, the source should see no net emf. The resistances of course are connected in series, so the source will see twice the static resistance and have to provide twice the Voltage to get the desired current. But you would have a Voltage and current which are completely unaffected by the magnetic field.

 

[Merlin3189]

And the physical arrangement would be like this attachment.

 

[Merlin3189]

And your diagram would be like the attached.

 

[XRZ]

Thank you @Merlin3189, I'll study this and get back to you.

 

[XRZ]

Well, the diagram that you used(of mine) seem to have increase opposition instead of canceling things.
Also this site my help you, free schematic site that allows you to save it to you computer, I used it to create that diagram.

 

[Merlin3189]

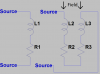
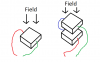
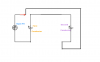
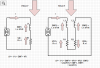
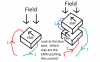
Thanks for the suggestion. I've used it for the attached diagram.

If there were any changes in the current I, then you would be correct that the reactance of both inductances would add in opposition to the change in the current. But if we are assuming the current is constant, then the only EMFs generated are a result of a change in the magnetic field. Since the magnetic field is in the opposite sense in each inductance, then the EMFs are in the opposite sense - as shown by the arrows. The PDFs generated in the (Ohmic) resistances always act to oppose the current (again shown by their arrows.)
So by having two slabs in the same magnetic field, but with the current passing in opposite directions, you get twice the resistance, but the EMFs generated by changes in the field oppose each other and exactly cancel out.
In practice of course it might be difficult to ensure the field through each slab is *exactly* the same, but it should be possible to get very close. Helmholtz (See http://en.wikipedia.org/wiki/Helmholtz_coil) realised this and devised a system of using two "ïdentical" coils, fixed symmetrically one on each side of the device under test. In your case, if the slabs are one on top of the other, the coils would be one above and one below, to make the whole apparatus symmetrical about a horizontal plane between the two slabs.

BTW I have not added many comments, but I have been trying to follow this thread and it has given me much pause for thought. Sometimes we think we understand something, until someone asks a "silly" question, or refuses to accept the "öbvious" answer, then you find yourself (or at least I do) analysing your ideas, trying to break them down into simpler steps to make a clear and convincing argument. That's when you find the gaps & flaws in your own understanding.
Although it's not germane to this question, trying to answer it has led me to Faraday's rotating disc paradox, which I had not met before.
http://en.wikipedia.org/wiki/Faraday_paradox
Also, though I think you simply cannot have a truly *constant* current generator, any more than you can have an irresistible force or an immoveable object, I am provoked to consider how I would calculate the response of a regulator to variations in load, particularly in the time domain. In the past I had just assumed that if I could plot the graph of the output for various steady loads and I put an array of capacitors (big ones for the LF and smaller ones for higher frequencies) then all would be well.
So whatever your reasons for asking, I think there is some value in trying to answer.

 

[Merlin3189]

And another annotation to a previous diagram to help see the cancellation of any magnetically induced EMFs.

 

[XRZ]

Thank you @Merlin3189, much appreciated.

 

[XRZ]

I was wondering about this diagram, if it would work.

Kind of the same idea, however, current would not flow from the PS to the second slab.