Thanks hevans, apologies if I'm starting to irritate you.
Not irritated at all, as you seem interested in actually
learning about electronics, instead of just blindly
copying what other people have done.
Learning about feedback was a hugely complicated effort for me in the 1960s because I didn't yet have either the math background or an understanding of the circuit theory I needed, nor a suitable instructor/mentor who could explain the concepts clearly. Negative feedback was the hardest because, in trying to analyze what was going on, I seemed to always be "chasing my tail" like a dog, instead of making any progress. By contrast, I thought positive feedback was literally a snap: the output was either on or it was off and positive feedback made sure that once it began to change from one state to the other it continued on that path without hesitation or oscillation in between. I did think this odd at first, since I "knew" that oscillation
required positive feedback. But I digress (as per usual)...
I am not a big fan of LTSpice simulation (or any other computer simulation) over hands-on experimentation with real components to learn electronics. A couple of 9V batteries and a 741-type op-amp, along with a handful of assorted resistor values and a cheap digital muiltimeter, will teach you pretty quickly what is going on with feedback, both positive and negative. Take careful notes of what you are doing so you can keep track of your learning progress. Here is a common-sense definition of feedback: applying a sample of the output back to the input to effect a desired response. With op-amps, you can take a portion of the output and apply that portion to either the inverting or non-inverting inputs, or both. Depending on exactly how you do this, the result can be either positive feedback or negative feedback.
There are several simplifying assumptions you can make about op-amps that will greatly speed circuit analysis, at the minor expense of some quantitative inaccuracy in the results. Once you have mastered elementary circuit analysis and design, you can use Spice programs to simulate circuits to "better" accuracy, but the results will be no better than the models used by the Spice program.
You will have to think about what the following simplifying assumptions mean in terms of their effect on circuit operation and analysis.
First, assume an op-amp has infinite differential gain for signals applied between the inverting and non-inverting inputs, that it's output can swing either positive or negative to any voltage, and that its output can supply any current to any load. Next, assume it can do this at any frequency without loss of response, that there is infinite impedance between the inverting and non-inverting inputs, and that if the same signal is applied simultaneously to both inputs (a common-mode signal input), there will be no change in output. This last property is called common-mode rejection. The ratio of output change to common-mode input change is called the common mode rejection ratio or CMRR and is a very desirable property of real op-amps, often being on the order of 100 db or more with careful design and construction.
The first assumption, infinite gain, means there
must be zero differential voltage between the inverting and non-inverting terminals for any finite output. Why? Because
any differential voltage, multiplied by infinite gain, results in infinite output voltage... not a practical outcome for real op-amps. For circuits with negative feedback, it is the feedback that forces the differential input voltage towards zero. For circuits with positive feedback, it is the power supply rails that determine how far the output voltage can actually swing, and that in turn affects the differential input voltage, which is not necessarily zero with positive feedback. In any case, we only pretend that the output can swing to plus or minus infinity voltage and then see what the consequences of the pretense would be in a real op-amp circuit.
Infinite impedance between the inverting and non-inverting inputs means zero current flows between those two inputs, so the only currents you need to be concerned with for circuit analysis are the currents through external components. No current enters or leaves the two op-amp input terminals. The ability of the output to provide any current to any load means you don't have to worry about the output voltage depending on output current or load resistance either, a fine if unrealistic convenience. Real op-amps have outputs that DO depend on their output current and the load resistance, but you can ignore that for purposes of a simplified circuit analysis.
Op-amps are often used as comparators, either because of ignorance or convenience. There are integrated circuits especially designed to function as highly accurate comparators with fast response times, avoiding most of the limitations in performance imposed by using an op-amp (basically a linear analog component) as a comparator (basically a digital component). Be aware that some comparators have open-collector (or open-drain) outputs, so the output state that occurs when the non-inverting input is more positive than the inverting input means the output is really "off" and must be pulled "high" with a resistor connected to the positive supply rail. Read the datasheet carefully to determine how the comparator output responds to signals applied to its inputs.
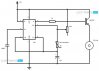
In your example CA3140 op-amp, used in your post #17 as a comparator
here, try adding a one meg-ohm resistor from the output on pin 6 to the non-inverting input on pin 3 to add some hysteresis. So, how much hysteresis does this add? Consider two states, output high and output low. Calculate the voltage at pin 3 for each of these states. The difference in the two voltages is the hysteresis and is a measure of how much the thermistor-derived voltage on pin 2 must change to produce an alternating change in output states. If you know the temperature-versus-resistance characteristics of the thermistor, you can relate this voltage change to a temperature change and hence a temperature hysteresis.
I suggest that you actually breadboard this circuit, using whatever op-amp you have on hand, and substituting a variable resistance for the NTC thermistor. Use your multimeter to investigate the hysteresis effect. You can leave out the transistor and relay if you want, connecting an LED with a current-limiting resistor directly to the output of the op-amp to provide a visual indication of the "on" and "off" states as you vary the resistance of the "simulated" NTC thermistor. Make careful notes of the voltage at pin 2 which causes the circuit to change states, and whether the voltage was increasing or decreasing when the state transition occurs. Try changing the one meg-ohm positive feedback resistor to higher and lower values to see what effect its value has on the switching point voltages. Try changing the set-point potentiometer connected to the other op-amp input to see what effect different settings have on the switching point voltages. After "playing" with this for an hour or so, you should have a pretty good idea of how hysteresis works.
But it burns me that I keep on finding circuits designed by people who know a helluva lot more about electronics than yours truly. Why have they not implemented hysteresis.

Why do you assume those people "know a helluva lot more than yours truly?" Last time I looked, there was absolutely NO qualification required to post ANYTHING on the Internet. The typical Instructables post is an excellent demonstration: although some are pretty good, some are plain awful, and many of them are full of errors and unwarranted assumptions. Most people have no idea what hysteresis is or how to use it.
If you want to learn about hysteresis, do some research that involves reading material from several different reliable sources so you can compare opinions and explanations. Basic circuit analysis texts may be of some help. Google is your friend, but trust nothing until you have verified its truth either from personal experiment or corroboration by other sources. Then hold fast and don't fall for spurious claims that deny what you have already learned to be true. This process takes many years to master, but it is never too late to learn.
Hysteresis is not always intentional. For example, it is the bane of position control systems (servo-mechanisms) where it causes positioning errors that depend on the approach direction. Expensive procedures, such as anti-backlash gear mechanisms, are often required to minimize it.
Hysteresis is unavoidable, and sometimes even desirable, in some applications. Most magnetic circuits exhibit magnetic hysteresis, and
the effect is sometimes exploited for purposes of heating: a hollow magnetic susceptor (typically made of nickel or a ferrous alloy) is placed in the work coil of an induction heater, where it will quickly become incandescent through induced eddy current losses and magnetic hysteresis losses as it heats the work object inside, indirectly, by infrared radiation.
OTOH, high-quality power transformers use
grain-oriented silicon steel to minimize hysteresis losses in the magnetic circuit. They must still use insulated laminated cores to minimize eddy-current losses, or use an insulated spiral tape core over which the windings are placed. A well-made transformer will have an efficiency of close of 100%, meaning almost no losses. There aren't too many other things made by man that can claim such good efficiency, although three-phase induction motors come close.
Please let me know if any of the above helped you, or whether it went flying over your head like a flaming meteor. Maybe I should have included some circuit diagrams...
Hop

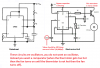
 Audiogurus's redesign
Audiogurus's redesign